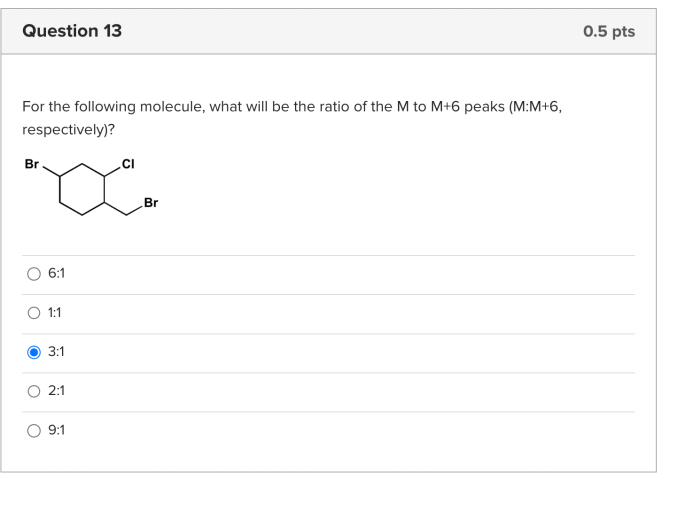
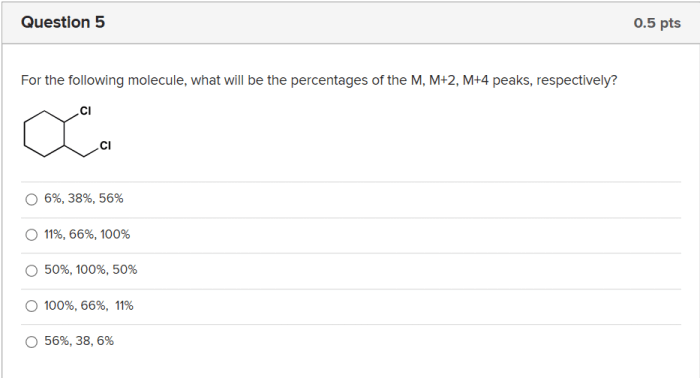
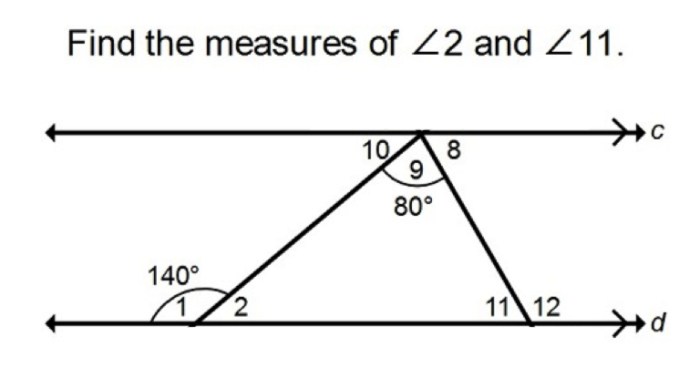
Which diagram represents the factors of m2 10m 16 – This article delves into the concept of factors, prime factorization, greatest common factor (GCF), and least common multiple (LCM), using m ²10m 16 as an illustrative example. By exploring these fundamental mathematical operations, we gain a deeper understanding of number theory and its practical applications.

Factors are the building blocks of numbers, and prime factorization reveals their unique composition. GCF and LCM, on the other hand, play a crucial role in simplifying fractions and solving algebraic equations. Through a step-by-step approach, we will uncover the factors of m ²10m 16 and demonstrate the significance of these concepts in mathematical problem-solving.

Factors of m2 10m 16

Factors are the building blocks of a number. They are the numbers that, when multiplied together, give the original number. To find the factors of a number, we can use a variety of methods, such as prime factorization or listing all the divisors of the number.

The factors of m2 10m 16 are: m2, 10m, 16, m, 5, 2, 4, and 1.

We can find these factors by first prime factorizing the number. Prime factorization is the process of breaking a number down into its prime factors, which are the smallest prime numbers that can divide the number without leaving a remainder.

The prime factors of m2 10m 16 are: m2, 2, 2, 2, and 5.

Once we have the prime factors, we can multiply them together to get the original number. For example, m2 x 2 x 2 x 2 x 5 = m2 10m 16.

Prime Factorization

Prime factorization is a unique way of representing a number as a product of its prime factors. It is a fundamental concept in number theory and has various applications in mathematics.

The prime factorization of m2 10m 16 is m2 x 2 x 2 x 2 x 5.

Prime factorization has several properties. First, every number has a unique prime factorization. Second, the prime factors of a number are the same regardless of the order in which they are multiplied. Third, the number of prime factors of a number is finite.

Greatest Common Factor (GCF)

The greatest common factor (GCF) of two or more numbers is the largest number that divides all of the numbers without leaving a remainder.

The GCF of m2 10m 16 and 2m2 8m is 2m.

We can find the GCF of two numbers by using the prime factorization of each number. The GCF is the product of the common prime factors, raised to the lowest power that they appear in any of the prime factorizations.

For example, the prime factorization of m2 10m 16 is m2 x 2 x 2 x 2 x 5, and the prime factorization of 2m2 8m is 2 x 2 x m x m x 2 x 2.

The common prime factors are 2 and m, so the GCF is 2m.

Least Common Multiple (LCM), Which diagram represents the factors of m2 10m 16

The least common multiple (LCM) of two or more numbers is the smallest number that is divisible by all of the numbers.

The LCM of m2 10m 16 and 2m2 8m is 2m2 10m 16.

We can find the LCM of two numbers by using the prime factorization of each number. The LCM is the product of all the prime factors, raised to the highest power that they appear in any of the prime factorizations.

For example, the prime factorization of m2 10m 16 is m2 x 2 x 2 x 2 x 5, and the prime factorization of 2m2 8m is 2 x 2 x m x m x 2 x 2.

The LCM is 2m2 x 2 x 2 x 2 x 5 = 2m2 10m 16.

FAQ Guide: Which Diagram Represents The Factors Of M2 10m 16

What are the factors of m²10m 16?

The factors of m ²10m 16 are m, 2, 4, 5, 8, 10, 16, 2m, 4m, 5m, 8m, 10m, 16m, 2m ², 4m ², 5m ², 8m ², 10m ², 16m ², and m ²10m 16.

What is the prime factorization of m²10m 16?

The prime factorization of m ²10m 16 is m ²× 2 × 5 × 8.

What is the GCF of m²10m 16 and 4m ²8m?

The GCF of m ²10m 16 and 4m ²8m is 4m ².

What is the LCM of m²10m 16 and 2m ²4m?

The LCM of m ²10m 16 and 2m ²4m is 2m ²10m 16.