Triangle sum theorem new years edition – The Triangle Sum Theorem: A New Year’s Edition delves into the fascinating world of geometry, exploring the versatile applications of this fundamental theorem in festive and educational contexts. From designing festive decorations to enhancing mathematical understanding, this exploration promises a captivating journey into the realm of triangles.
Unveiling the essence of the Triangle Sum Theorem, we embark on a quest to unravel its historical significance, tracing its origins and acknowledging the contributions of notable mathematicians. Through interactive activities and thought-provoking examples, we illuminate the theorem’s impact on art, design, and cultural traditions.
Triangle Sum Theorem

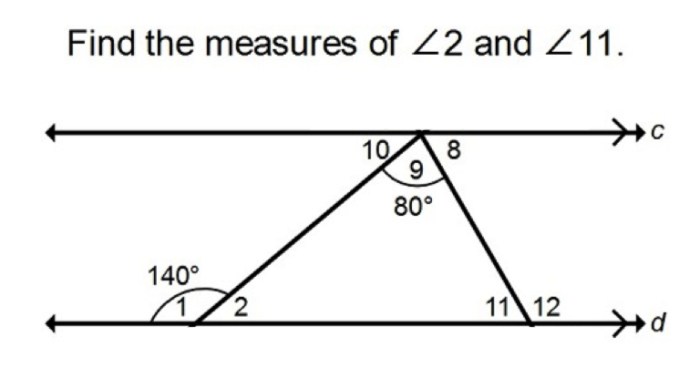
The Triangle Sum Theorem states that the sum of the interior angles of a triangle is always 180 degrees. This theorem is fundamental in geometry and has numerous applications in various fields.
Examples of Triangle Sum Theorem Usage in Geometry, Triangle sum theorem new years edition
- Determining the missing angle in a triangle
- Calculating the area of a triangle
- Proving triangle congruence
Triangle Types and Corresponding Angle Sums
| Triangle Type | Sum of Angles |
|---|---|
| Equilateral Triangle | 180° |
| Isosceles Triangle | 180° |
| Scalene Triangle | 180° |
| Right Triangle | 180° |
New Year’s Edition

Applying Triangle Sum Theorem to New Year’s Activities
The Triangle Sum Theorem can be incorporated into New Year’s celebrations in creative ways:
- Designing festive decorations with triangular shapes
- Creating party games that involve calculating triangle angles
- Using the theorem to enhance the understanding and appreciation of New Year’s traditions
Applications in Art and Design
Triangle Sum Theorem in Various Art Forms
The Triangle Sum Theorem is widely utilized in art and design:
- Painting: Composition and perspective
- Architecture: Structural stability and aesthetics
- Graphic Design: Logos, typography, and layouts
Famous Artworks Incorporating Triangle Sum Theorem
Examples of renowned artworks that exemplify the theorem’s application:
- Leonardo da Vinci’s “Mona Lisa”
- Vincent van Gogh’s “Starry Night”
- Piet Mondrian’s “Composition with Red, Blue, and Yellow”
Historical Significance: Triangle Sum Theorem New Years Edition
Origins and Development of Triangle Sum Theorem
A historical overview of the theorem’s evolution:
- Ancient Egypt: Empirical observations
- Euclid’s “Elements”: Formal proof
- 19th century: Extensions and generalizations
Key Mathematicians and Their Contributions
Notable individuals who advanced the theorem’s understanding:
- Euclid
- Carl Friedrich Gauss
- Bernhard Riemann
Educational Implications
Triangle Sum Theorem in School Curricula
The theorem’s significance in mathematical education:
- Understanding angle relationships
- Developing problem-solving skills
- Preparing students for higher-level geometry
Interactive Learning Activities
Engaging activities to enhance student comprehension:
- Hands-on experiments with triangles
- Interactive simulations and games
- Problem-solving challenges
Cultural Connections
Triangle Sum Theorem in Cultural Traditions
Examples of cultural practices influenced by the theorem:
- Chinese architecture: Feng shui and geomancy
- Islamic art: Geometric patterns and mosaics
- Native American crafts: Beadwork and pottery
Triangle Sum Theorem in Artistic Expressions
The theorem’s impact on various artistic forms:
- Indigenous art: Symbolism and spirituality
- Modern art: Abstract compositions and sculptures
- Photography: Perspective and composition
FAQ Explained
What is the Triangle Sum Theorem?
The Triangle Sum Theorem states that the sum of the interior angles of a triangle is always 180 degrees.
How can the Triangle Sum Theorem be used in New Year’s decorations?
The Triangle Sum Theorem can be used to design festive decorations such as garlands, ornaments, and snowflakes, ensuring symmetrical and visually appealing shapes.
What are some examples of famous artworks that incorporate the Triangle Sum Theorem?
The Triangle Sum Theorem is evident in the composition of paintings by renowned artists such as Leonardo da Vinci and Piet Mondrian, adding structural balance and harmony to their masterpieces.